In the quest to understand the complexities of shapes defined by mathematical equations, the Algebra II Worksheet on Parabola, Circle, and Ellipse emerges as a comprehensive tool intended to guide students through a variety of scenarios involving these curves. It begins by diving into the world of parabolas, prompting learners to find the focus and directrix of given parabolas, which are crucial components in their definitions and properties. The worksheet then expands the challenge by asking for the graphing of these parabolas, including critical features such as the vertex, focus, directrix, and additional points to give a fuller depiction of the shape. Moving beyond parabolas, the worksheet shifts focus towards circles, leading students through exercises that involve sketching these and identifying key elements like the center and points on the circumference. The journey through geometric figures continues with ellipses, where students are tasked with determining foci, vertices, and covertices, alongside sketching and composing equations to represent these ellipses accurately. Furthermore, the worksheet prompts a comparative analysis between the equations of ellipses and circles, fostering a deeper understanding of their similarities and differences. This array of exercises is meticulously designed not only to test the mathematical prowess of students but also to enhance their appreciation of the elegant interplay between algebra and geometry as manifested in the study of parabolas, circles, and ellipses.
| Question | Answer |
|---|---|
| Form Name | Parabola Worksheet Form |
| Form Length | 4 pages |
| Fillable? | No |
| Fillable fields | 0 |
| Avg. time to fill out | 1 min |
| Other names | homework parts of a parabola answer key, 10 3 features of a parabola answer key, parts of a parabola worksheet answer key, key features of a parabola worksheet answers |
Algebra II Worksheet Parabola, Circle, and Ellipse |
Name_____________________________________ |
Period________
1.Find the focus of the parabola:
2.Identify the focus and directrix of the parabola given by
3.Identify the focus and directrix of the parabola given by
4.Graph the parabola. Include the vertex, focus, directrix, and four points other than the vertex.
5.Write the standard form of the equation of the parabola with its vertex at (0, 0) and focus at (0,
6.Write the standard form of the equation of the parabola with its vertex at (0, 0) and directrix .
7.Write the standard form of the equation of the parabola with its vertex at (0, 0) and directrix
8.Suppose a parabola has vertex and the distance from the vertex to the focus is 5 units. How many possible parabolas fit this description? Write the equations of all the possible parabolas that fit this description.
9. Sketch the graph of X2 + Y2 = 49 . Give the center and 4 points on the circle.
10.Write the standard form of the equation of the circle with radius 6 and center at (0, 0)
11.Sketch the graph of . Give the center and 4 points on the circle.
12.Write the standard form of the equation of the circle that passes through the point (0, 1) with its center at the origin.
13.Write the standard form of the equation of the circle that passes through the point (3, 4) with its center at the origin.
14.Write the standard form of the equation of the circle that passes through the point (1,
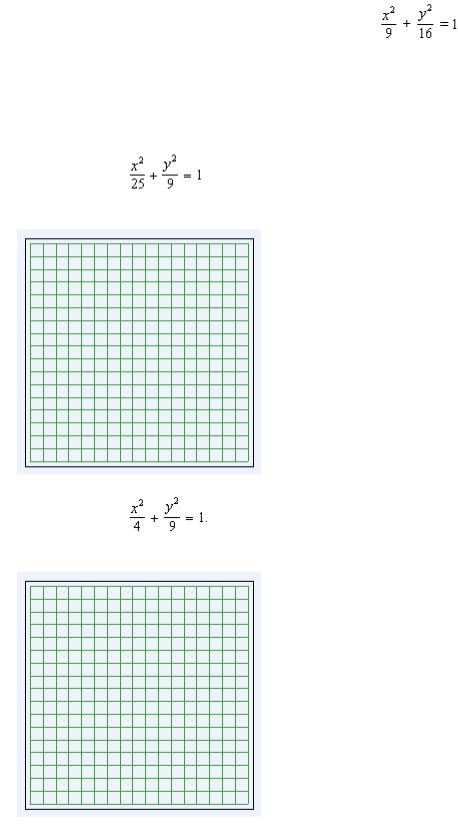
15. Determine the foci, vertices, and covertices of the graph of
16. Sketch the graph of |
. Include the vertices, covertices,and foci. |
17. Sketch the graph of |
Include the vertices, covertices,and foci. |
18. Sketch the graph of . Include the vertices, covertices,and foci.
19. Write an equation of an ellipse with vertices of
20.Write an equation of the ellipse with a vertex at (9, 0), a
21.Write an equation of the ellipse with a vertex at (0, 8), a
22.Write an equation of the ellipse with a vertex at (5, 0), a focus at (4, 0), and center at (0, 0).
23.Writing: How is the equation of an ellipse like the equation of a circle? How are the equations different?