Understanding the intricacies of documentation that bridges the landscape of legal and scholarly requirements is essential, especially when such documents encapsulate a wealth of information within complex systems of coding and signification. The Igi5 form, as documented in the volume 7, no. 4, 1997 edition, spanning pages 393-407, stands as a quintessential example of such a phenomenon. This form not only navigates through the dense foliage of academic reporting and legal documentation but also encapsulates a myriad of symbols, each bearing distinct significances that range from operational codes and procedural indices to annotations that underline scholarly commentaries and legal precedents. It operates within a multifaceted realm where the academic meets the legal, overlaying multiple layers of information through a complex symbology that demands not just a cursory glance but a deep, analytical and interpretative approach to fully comprehend its utility and application. Such documents underscore the necessity of cross-disciplinary literacy, inviting professionals not just to decode but also to engage with the material in a manner that respects its depth and breadth. The exploration of the Igi5 form serves as a gateway into understanding how specialized forms of knowledge are organized, communicated, and preserved within legal and academic frameworks, highlighting the ongoing dialogue between these spheres to facilitate information exchange and knowledge dissemination.
| Question | Answer |
|---|---|
| Form Name | Igi5 Form |
| Form Length | 15 pages |
| Fillable? | No |
| Fillable fields | 0 |
| Avg. time to fill out | 3 min 45 sec |
| Other names | project igi 5 full game no No Download Needed needed for pc, igi5 how mb, igi5 No Download Needed for computer, igi 5 no No Download Needed needed |
|
|
|
|
|
|
vol. 7, no. 4, 1997, pp. |
|
||
✚✜✛✌☛✞✄☞✝✣✢✥✤✦✌✡✑✟✠✡✒✍✘☞✑✟✧✔✌★ |
|
|
|
||||||
|
✷✹✸✻✺✼✸✻✽✿✾❁❀❃❂✗❄❆❅❈❇❉❄✍❇❊✺✼✺✻❋✴✺❍●❈■✪❋✴❏▲❑✿▼✞✸✻❂◆✽ |
|
|||||||
|
|
|
|
|
❜✖❝✆❞✻❡❢❝✏❣✐❤❍❥✱❤❧❦✡♠❴❡❢♥♣♦❨❦✡ |
|
|||
|
|
|
|
|
|
⑥✉⑦✉⑧✏⑨▲⑩❶✉❷◆❷❉❸❺❹❼❻✆❽✉❾❿⑧✉➀✍❹✻⑦♣➁▲➂✪➃♣❹☞➄❢➆⑨✦➇❺➄✟➈❃➉✉➊ |
|||
|
|
|
|
➑➈✆➀✍❹❃❹✏➈♣⑩⑧☎➈♣➅✯➒➓❶✉⑩❽✉➌❺➈❃➃☞➔✞➀✍➇➐➃➣→✏➀✍❹✈➌❺➇➐➌↔➊✱➌➆➈✏❸❧↕✍➅✼❶✉❽✙❸◆➙➣➄✈➛✗➄✗➜✉➝✵➜☎➞✞➟✡➠✼➡☎➢☎➤✞➟♣❸★➥✴➏ |
|||||
|
|
|
|
|
|
➋➈✼⑨✱⑧✉➅✏➌❺❾❍➈♣➀✍➌✪❶✻➍③➎✗❶✉❾✯⑨✦➊✱➌❺➈♣ |
❧➏✭➃♣➇➐➈♣➀✦➃❃➈ |
||
|
✌✡✠✙✑✣➩✱✠✉✢➭➫❺✄☞✠✡✌✙✠✡✑✟➩❴✠✉✢❚✢✭✁✵✘☞✠☎➯ |
|
|
||||||
➧➨✠✡➩✦✑✟✎✏✠✉✢➲➫❺✄☞✠✡➩✦✑✟✎✏✠✉✢❪✢✔✁✵✘☞✠✉➯ |
|
|
|
||||||
✮✯☛✞✰✳✰➳✩✭✒✔✑✟✌✉✁✵✘☞✠✡✢✖✫✍✶❆➫➆➵❿✁✵✰✳✠❿☛✞➸✪➺➓✢✭✑❢✘☞☛✵✄♣➯ |
|
||||||||
|
|
|
|
|
|
➻➨➼➣➽➚➾◆➪➨➻❛➶➣➾ |
|
|
|
|
|
|
➹➘✑✟✝✣✑✟✒✭✓❈✬✔✁✞✎➓✫❉✠✡✠✡✒✳✩✔✎✏✠ |
|
|
|
|||
|
✌✡☛✱✰✳✲✭✑✟✝✣✠♣➷✼ |
✑✟✰✳✠★✘☞✠✡✌✆✬✔✒✭✑➐❐✍✩✭✠✱❒✴✌✉✁✵✝✟✝✣✠✡✢❆✰✳✑✟✎✏✎❃➷✻✢▲✄☞✑✟➩❴✠✡✥✌✡✁✞✌✆✬✔✠❛✎✏✑✣✰❈ |
|||||||
|
✘✈✃✯✠✡✠✡✒✳✘☞✬✔✠➣✭✄☞☛✍✌✙✠✡✎✏✎✏☛✞✄✉✎❧❉✠✉✁✵➬✐✑✟✒✔✎❃✘✏✄☞✩✔✌♣✘☞✑✣☛✞✒✳✄✆✁✵✘☞✠❿✁✵✒✴✢➳✘☞✬✭✠❮✰✹✁✵✣✒✳✰✳✠✙✰✳☛✞✄✏✶➳✁✞✌❈✁✞✒✴✢❆✘☞☛❚☛✱✲▲✘☞✑✣✰✳✑✟➴✢✐✫✦✶❈✲✴✁☎✄✆✁✞✝✟✝✟✠✡✝✟✑✟➴✡✑✟✒✔✓➳✌✙☛✱✰✳✲✔✑✟✝✟✠✙✄☞✎✪✘☞☛❛✢✭✠✙✧ |
||||||||
|
|
✆✁✞✎✏➬✦ |
|
|
✳✌✡✁✞✌✆✬✔✠✹✲❉✠✙✄✏➸❺☛✵✄☞✰✹✁✞✒✭✌✡✠✱➮❚➱✻➲✘☞✬✔✑✟✎③✲✴✁✵✲❉✠✙ |
||||
|
|
☞✑✟✝✣✠❛✎✏✑✟➴✡✠③✬✴✁✵✘❿✁✞✌✆✬✔✑✟✠✡➩❴✠✙✎❮✘☞✬✔✠③✬✭✑✣✓✞✬✔✠✡✎❃✘❍✌✉✁✞✌✆✬✔✠❛✬✔❢✘✏➷❼✄✆✁✵✘☞✠✞➮❍➹➘✬✭✠ |
|||||||
|
➸❺☛✵✄❮☛☎➩❴♣✄✁✞✝✟✝➚✲▲✄☞☛✱✓✞✄✆✁✵✰ |
|
|
|
|||||
|
✶▲✎✏✑✣✎➳☛✞➸❍✁❆✝✟☛✍☛✱✲❨✒✔✠✙✎❃✘✹✁✞✒✔✢❩✘☞✬✭✠✡✒✌✉✁✞✌✆✬✔✠❧✰✳☛✦✢▲✠✡✝➆➮✂ |
|
|||||||
|
✑✟✒➭✰✳☛✦✢▲✠✙✄☞✒✩✭✄✂✘☞✡✌✬✔✒✭✑✣❐✦✩✭✠➘✑✣✢✭✠✡✒✍✘☞✑❢✧✴✠✙✎➓✲❉☛✵✘☞✠✡✒✍✘☞✑✣✁✞✝✔✌✡✁✞✌✆✬✔✠❧✰✳✑✟✎✏✎✏✠✡✎✪✘☞✬✭✄☞☛✱✩✭ |
||||||||
|
☛✱✒✔✢❈☛✱✒❈✃➘☛✞✄☞➬✦✎❃✘✆✁☎✘☞✑✣☛✞✒✔✎✡❒✍✃➣✑❢✘☞✬✐✎❃✘✆✁☎✘☞✑✣✎❃✑✟✌✡✎✿☛✞➸❊✰✑✟✎✏✎✏✠✡✎✪✲❉✠✙✄✪✄☞✠✙➸↔✠✙✁★✲✭✄☞✱✓✵✄✆✁✞✰ |
||||||||
|
|
|
✛✠➣✢▲✑✣✎✏✌✙✩✔✎✏✎✂✘☞✬✔✠➘✄☞✠✡✎✏✩✭✝❢✘☞✎✿☛✵➸✗✁✞✲✔✲✭❢✶✭✑✟✒✭✓❍✬✔✑✟✪✰✳✠✙✘☞✬✔☛✦✢③✘☞☛❿✓✱✩✭✑✣✢✭✠ |
||||||
|
|
|
|
|
|
|
✲▲✘☞✑✣✰✳✑✟➴✉✁☎✘☞✑✟☛✱✒◆❒✔➹➘✑✟✝✟✑✣✒✭✓✭➮ |
||
|
|
✟✒✦✘☞✠♣✄✏➸❺✠✙✄☞✠✡✒✭✌✡✠★✌✡☛✞✩✔✒✍✘☞✎➣✓❴✁✵✘☞✬✭✠✙✄☞✠✉✢❚✁☎✘❧ |
|||||||
|
✑❢✘☞✠✙✄✆✁✵✘☞✑✟☛✱✒❚➸↔☛✞✄➨➩✞✁✵✄☞✑✟☛✱✩✭✎➨✌✡✁✞✌✆✬✔✠★✲✴ ✄✆✁✞✰✳✠✙✬✔✠★✎☞✁✵✰✳✠❍✘☞✑✟✰✳✠✱➮✠♣✄☞✡➮ |
||||||||
|
✌✡☛✱✰✳✰✳☛✞✒✔✝❢✶❩✩✭✎✏✠✉✢❵✌✡☛✞✰✳✲✔✩▲✘✆✁✵✘☞✑✟☛✱✒✔✁✞➣➬❴✠✙✄☞✒✭✠✡✝✟✎✹✁✞✎✹✰✹✁✵✘✏✄☞✑❢ |
||||||||
✎❮ |
✁❍✽ |
|
✠✟✏ |
✝✆ |
✄✂❿ |
☎★ |
✞✆ |
☛✡☞✍✌✈ |
|
✑✏✔ |
|
||||||||

|
✏✟ |
|
✁◆ |
|
|
|
|
|
|
✄✂✴ |
✆☎✞✝✠✟✡✝✠☛✌☞➭ |
✍✂✴ |
|
|
❿✂ |
|
||
|
❿✂ |
|
|
★ |
❃✟✏✂▲ |
|
|
|
|
|
✍✂❉✎❊ |
|
|
|
|
|
|
|
✑✓✒✠✑✕✔✗✖✙✘✝✖✙✚✜✛✣✢✥✤✝✠✟✡✝✠☛✦☞✢✧✛✩★✫✪✧✬✮✭✯★✰✛✩✘✝✠☛✌☞✥✱✖✧✬✲✛✩★✴✳✵✔✗✖✶✢✧✖✧★✰✖☛✸✷✖✺✹✻✛✷✍✼✩✟✡✝✠☎ |
|
|
|
|
✁✿✡✞✌ |
|||||||||||||
✽ |
|
|
✧✾❉ |
|
|
✔✏ |
✔✏ |
|
|
✔✏ |
|
✧✾❉ |
✺✗ |
|
|
|
|
|
★ |
❃ |
✧✾❉ |
|
|
|
|
|
☛❿ |
|
|
|
|
❿✂ |
|
||||
|
✁❮ |
|
❀✂✭★ |
|
|
|
|
✁✿✓✿✡✭✁✿❂❁➘✌ |
✙✾❉ |
|
|
|||||||
|
|
|
|
|
❆❅✖❇✒✦☞ |
|
❃✺❈✮❉✗❊ |
|
❄✂✭ |
|
|
❍●✉ |
||||||
❍✎ |
✧■ |
|
|
|
|
❮ |
✺❋❈✗❉✗❊ |
|
✁❍ |
|||||||||
|
▼❏ |
|
❇❏ |
|
|
❇❑❍ |
❖◆☞ |
|
|
|
||||||||
|
❮✎ |
|
|
✙✾❉ |
|
✁ |
|
✍✂✭ |
|
|
▲◆ |
❘■➚ |
|
|||||
|
❿✂ |
✡✗✁✌◗❃✏✟ |
❿✂✡✁✌◗❿ |
|
|
|
|
★ |
❃✟ ✭✏✁◆ |
|
|
|||||||
✑✓✒❚❙❯✼❱✷❳❲ |
|
✼✩☛✸✷✖❪✤❫✖✷✍❲✦☛❫✝❵❴✧❛✧❜ |
|
|
|
|
▲✗ |
|
||||||||||
✂❿❮❝✗ |
|
✖❩❨❬✖✙★❭✢✧✛✩★✴✬❍ |
✖❳❞✦✖✷✙❛❖☎✞✝✛☛❢❡❀❣★✝✘❂✖✗☛ |
❜✴✳✩✬✏❤✍✛✟✡✝❵✷☎ |
|
|
|
|||||||||||
|
✐✙❥➆❵❦✞❧✶♠❂♦❦✈⑤❢♥✉❄♥✆❦✱❝❮❝✍♥ ♦▲❝✠✂✣♥✣❦❿✻⑤☎❝☞ |
|
✍❦✯ |
✶♥✣♠❴❞✈❞✻❝✆✫♣❴❝✣❧✈❝✉❥ |
|
|
|
|
|
|||||||||
|
|
|
|
|
|
|
|
|
|
❁ |
|
|
|
|
|
|
|
|
❿✂ |
|
✧✾❉ |
✙■➚ |
★ |
|
|
|
|
❿✂ |
|
|
|
|
❿✂ |
✡✌✈ |
|
|
❿✂ |
|
|
|
|
✁❍ |
|
|
★ |
|
❂✾✴ |
|
|
|
✧✾❉ |
|
||
|
|
|
✡❉✁✁✌✂❉ |
|
❮ |
|
|
✆ |
✡✗◗✗☞✁✌✄❁ ★✧✾❉ |
|
|||||||
✻❏ |
|
|
❿✂ |
★ |
❊ |
|
|
|
|
|
|
|
❍✂ |
|
|||
✏✟ |
|
✄✡❃❮✌ |
|
|
|
|
|
|
|
|
|
|
|
||||
|
|
|
❍ |
|
|
|
✧✾❉ |
❿✂ |
|
|
❿✂ |
|
|
||||
|
|
|
❃✟ |
|
|
|
|
|
❮ |
|
|
|
|||||
★❿✂ |
|
|
|
|
|
|
★ |
|
|
✁❮ |
|
✡✌✈ |
❉ |
▲◆ |
|
||
★ |
|
★ |
|
✧✾✴ |
|
|
❿✂ |
✧✾❉★ |
|
|
|
||||||
|
|
|
|
|
★ |
★ |
☞◆ |
❉ |
✙✾❉ |
❮✎ |
✑✏✔ |
|
✻❏ |
||||
❍☎ |
|
|
|
|
|
✓✂✭ |
★ |
|
✄✂❿✧■ |
|
|||||||
|
|
|
|
❜✙✖✈☎ |
|
✁✾➳ |
❿✂ |
|
❘✂✭✝✆✭ |
|
✡✠❱ |
|
|||||
❿✂ |
|
|
|
|
☞☛✟✞❱③ |
|
|
|
✁③ |
❆✟✡✝✠☛✖ |
|
|
|
||||
|
|
|
|
|
|
|
|
|
|
✴❋ |
|
❉❁✵❋✴ |
☛☞➭ ✁③ |
|
|
||
|
|
|
|
|
|
|
|
|
☞ |
❮ |
|
✍✌✯ |
✺❋✴ |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|

|
✁✄✂☎✂✆✟✞✸ ❆❄✍✸✡✠➚❋✴✽❭❪❇❊❏❴❫✯❋➓✸✼❯❁❑✿✺✻❇❉▼✞✸✻❂◆✽ |
|
|
|
|
|
✧✾❉ |
★★ |
|
|
|||||||||||||||||||||||||
❉❙✌✒✠✑❨★✰✖✟✡✝✬✝✠☛✯✼★✴✳✍✌❆✖✗✌ ✏✎☛❫✝✠☎✶✝✛❜☞☛ |
|
|
|
|
|
|
★ |
|
|
|
|
★ |
|
|
|
|
|
|
|
|
|
|
|
||||||||||||
❈ |
✧✾❚ |
▲■✁✗✁✗❿ |
|
✧✾✔✏ |
✧✾ |
|
|
|
|
|
✧✾❉❿✂ |
★ |
|
|
|
▲◆ |
❿✂ |
|
|
||||||||||||||||
★★ |
|
|
|
|
|
|
|
|
|
|
|
|
|||||||||||||||||||||||
|
❿✂✧✾✴ ▲✗ |
|
✁★ ✧✾❉ |
✧■❿✂ |
✒✑❮ |
✏❅✧✾✻✴ |
|
✡✌✠●☎ |
|
|
|
|
|
|
✁✗✙✾❉ |
|
✁★✗ |
❇❏ |
|||||||||||||||||
|
▼✿❪ ✁③ |
|
✙✾❉ |
✴❋ |
|
|
|
|
|
|
|
|
|
|
|
|
✁✗ |
|
|
|
|
|
|
|
|
|
|
|
|
|
✯✌ |
||||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
||
✓✡✔✖✕✍❁✘✗✚✙✛✜✿✈✌ |
❿✂ |
|
|
|
|
|
|
|
|
|
|
|
|
❿✂ |
|
|
|
|
|
|
|
|
|
✿◗✁➲✗ |
|
|
|
|
|
▲➚ |
|||||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
||||||||||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|||||||||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|||||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
||||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
❉ |
|
|
❊⑤✢✤✣❴❥✦✥★✧✪✩✖✫❴❦✡②★♠✱❡❢❝❥✈❝✣❧✈❦❳❦✈⑤❢♥✉✍♥☞❝✭✬➐♥✴❧✯✮✱✰✲✴✳✏✵✯✶✸✷✺✹✻✥✽✼ |
|
|
|||||||||||||||||||||||||||||
|
|
|
|
|
❿✂ |
|
|
|
❍ |
|
|
✍✂✭ |
❆☞✓❛❢✼★③❣ |
|
|
|
✿✂ |
|
|
❙✌✒❚❙✝✬❛❖✟✁✼✩☎✞✝✛✂✁☛✫✟☞✛✩★✝✠☎❭❲✬ |
|
|
|
|
|
|
|
|
|
|
|
|
|||||||
❿✂ |
|
|
|
|
|
✁★ |
❆☞✓✟✛✓❤✼❘✟❫✧✟✛✷☎✄✍❿✂ |
|
|
|
|
❍✯✌ |
|
|
|||||
❿✂❉❁❉❁✥❚✿◆❮✎ |
|
|
❉ |
|
|
✝✆❧ |
|
▲✗✿✂ |
|
|
|
|
✄❁❚ ✁③ |
||||||
✟✥ |
✧✾❊ |
☞☛✍✏✎✍✑✍✒✔✓✖✕✘✗✝✙✚✞✡✠☞☛✍✌✏✎✍✑✍✒✔✓✜✛☎✗✢✙✤✣✡✠☞☛✍✌✏✎✍✑✍✒✔✓✖✕✘✗✢✙✥✣✡✠☞☛✍✌✏✎✍✑✍✒✔✓✜✛☎✗✝✙✤✛✧✦☎★✍✩ |
|||||||||||||||||
✪✵ |
✞ |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
✰✣✡✠✬✫✭✌✭✮✏✒✚✙✂✕✯✦✢✛✧✧✦✧✦✧✦✧✦ |
|
|
|
|
|
|
|
|
|
||||||||||
✱✳✲✭✴✏☛✥✵✤✙✶✕✸✷✹✕✯✦✧✛☎★ |
|
|
|
|
|
|
|
|
|
|
|||||||||
✺ |
|
✲✭✴✏☛✂✻✼✙✚✛✽✷✾✛✧✿✧❀✽❁❃❂❄✙✢✛✽✷✾✛✧✿✧❀ |
|
|
|
|
|
|
|||||||||||
❅ |
|
✒✏✎✍❏✞✡✓✬✵✡✷❆✻✘✗✧✙✧✣✡✓✬✵✍❇❈✕✸✷❆✻✘✗✧❇✧✣✡✓✖✻❊❉❋✕✸✷●❂✍❉❍✕✘✗✧❇✍✣✡✓✖✻✘❇■✕✸✷●❂✍✗✧❇✧✣✡✓❆✻✸✷●❂✧❇❈✕✘✗ |
|||||||||||||||||
❑ |
|
✲✭✴✏☛✤☛✚✙✶✕✸✷✾✛✧✿✧❀✽❁▲✮▼✙✂✕✸✷✾✛✧✿✧❀ |
|
|
|
|
|
|
|||||||||||
◆✯◗✾✒✏✎✍❏✥ ✣✡✓☞☛✔✷❖✮❊✗✧✙✧✞✡✓☞☛✔✷❖✮❊✗ |
|
|
|
|
|
|
|
|
|||||||||||
✴❋ |
❿✂ |
|
❍ |
✯✌ |
|
|
❊⑤✢✤✣❴❥✵☎✧ |
❝❅✼ |
✓■❴❡✟❦♠❳❦✻⑤❢♥✉✴♠❂❧✞♥☞❝✭✢❊⑤❢❡❢❝✧✗ |
❼❅ |
|
✿❇●★ |
✁✗ |
||||||
|
|
|
|
❘■✂✙✗ |
|
|
|
✁③ |
❘✾➲ |
➳● |
✼❅ |
✵❁ |
|
✌●☞★ |
|
||||
|
✔❋ |
|
❮❿✂ |
|
|
✭✏ |
❤✼❜✙✖ |
|
✁★ |
✄☞ |
|||||||||
|
|
|
|
✧✾❊ |
|
✯✌ |
❉❁❙❘❛ |
|
✁★ |
❩✂▲ |
|
|
|
✿✌ |
|||||
|
|
|
|
|
|
|
|
|
|
|
|
✫✗ |
|
||||||
|
|
|
|
|
|
✠✂❿ |
|
|
▲◆❿✂ |
|
✁ |
|
|
|
|
|
|||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|

|
i |
[min,max] |
|
j |
[min,max] |
|
|
|
|
|
1 |
Loop |
1 |
|
Loop |
1 |
Assignment |
|
|
|
|
|
|
|
|
|
|||
|
|
[k,i] |
|
|
[k,i,j] |
2 |
3 |
4 |
5 |
|
|
|
|
|
1 |
||||
1 |
k [min,max] |
|
|
|
A[i,j] |
B[i+1,j] |
B[i,j+1] |
|
B[i+1,j] |
Program |
Loop |
|
|
|
|
||||
|
|
|
|
|
|
|
|
||
|
1 |
|
|
|
|
|
|
|
|
|
[k] |
|
|
|
|
|
|
|
|
|
r |
[min,max] |
|
s |
[min,max] |
|
|
|
|
|
2 |
Loop |
|
|
Loop |
|
Assignment |
|
|
|
|
1 |
|
1 |
|
||||
|
|
|
|
|
|
|
|
||
|
|
[k,r] |
|
|
[k,r,s] |
1 |
2 |
|
|
B[r,s]
A[r,s]
❿✂❉❁❉❁❁ ❮✎ |
✝✆❆✏✟ |
|
|
❊⑤✢✤✣❴❥✰✧✪✩✖✫❴❦✡②★♠✱❡❢❝✁✯ ✍❦❃❦❳❦✻❝☞✄✂◆❦✍❧✻❞✈❝✁☎❖❧✻❝☞❝ |
|
|
||||||
❿✂ |
➣✑ |
▼❋✴ |
✁✗ |
✧✾❃✴ |
✲❋✔ |
|
✆❁❊✌●☞✉ |
✧✾✴ |
||||
✏✟ |
|
|
|
|
✟✞✡✠☞☛✍✌✏✎✒✑✔✓❍◆☞ ❪❅✶❋❉ |
|||||||
|
|
Insert |
|
Next |
NextCand |
Global Clock |
|
|
|
|
||
|
|
Event |
|
Event |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
NextLex |
|
|
|
|
|
✿✌❉❁❉❁☞ |
❊❁❼❅✠●❿✁③✴❋[1,1,1,1,10,1,2,1,4][NextCand]✁★ ✖✕✽✗✁✗✆✄❅❼✠●③ |
|
✧✾✴ |
|
❅✠●❼❛ |
☎✂❿ |
|
|
||||
|
|
|
|
|
|
|
Miss Processing |
Miss |
Cache |
|
|
|
|
|
|
|
|
|
|
Simulation Loop |
|
|
|||
|
|
|
|
|
|
|
Data |
Probe |
|
|
||
|
|
[1,1,1,1,10,1,33,1,3][NextCand] |
|
|
|
|
|
|||||
|
|
|
|
|
|
|
|
|
||||
|
|
[1,1,1,1,10,1,34,1,2][NextCand] |
Affected |
|
|
|
|
|
||||
|
|
|
|
|
|
|
|
|
|
|
||
|
|
|
|
|
|
Event |
|
|
|
|
|
|
|
|
[1,1,1,1,33,1,2,1,1][NextCand] |
Update |
|
|
|
|
|
|
|||
|
|
[1,1,1,1,33,1,2,1,5][NextCand] |
|
|
|
|
|
|
|
|||
|
|
[1,1,1,2,1,1,1,1,1][NextCand] |
Event |
|
|
|
|
|
|
|||
|
|
|
|
|
|
Guards |
|
|
|
|
|
|
|
|
[1,1,1,2,1,1,1,1,2][NextCand] |
|
|
|
Guarded Cache Model |
|
|||||
|
|
|
|
|
|
|
|
|
|
|||
|
|
|
|
Sorted Event List |
|
|
|
|
|
|
|
|
✂❿ |
▲✗ |
❖◆☞✢✤✣❊⑤❴❥❈✱✧✾✴✧✖✩❉❫☎❝☞✧❦✙✘✴⑤❢❞❵❦➓❦✡✏✔✑➨✛✚♠✍❧✍❦✻ |
✁✄✜✗✂❦✍♥❴❝✣✢❉✐♥✵ |
✏✟ ✧✾❉ |
|
✂ |
|
|
|
|
|
|
|
|
|
❿✂ |
|
|
☛ |
|
|
|
❖◆❿✂▲■☞ |
|
③✞ |
❖❊✌ ✗ |
|
❿ |
|
✄❅✁✠ |
✿❇● |
▲■❱✠ |
||||
|
|
✿✌ |
|
✙✗✁ |
❿✂ ✁③ |
|
|
|
❘✂▲ ❘■ |
✆❅✽❈✄✂✳● |
✧✾✴ |
|
▲◆ |
|
|
|
☎★ |
|
||||
✿✌ |
|
|
|
✍✂ |
|
|
|
|
|
|
|
▲◆✏✟ |
|
|||||||||
|
❿✂ |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|||
|
✭✏ |
|
|
|
|
|
▲◆ |
|
|
|
❿✂✝☎✞✡☛✑✔✞✗✠☛❛ |
|
|
✙✾✔ |
|
✧✾✴ |
|
|||||
|
❿✂ |
|
|
|
✓■✟✞✡✠☞☛✍✌✏✎✒✑✔✓✆✆☎✞✗☛✑✔✞✡✠☛✯ |
|
|
|
|
|||||||||||||
|
|
✞☎✍✂✥✞✡☛✑✔✞✗✠☛✳ |
❍ |
|
❿ |
❍❿✂ |
|
▲◆ |
|
❩❅✼ |
|
|
|
❳●☎❿✂ |
✑✏✔ |
|||||||
|
✁❍❃✟ |
✁✗ |
|
|
|
|
|
✄✂❍❿ |
|
|
|
❍ |
❬✗ |
|||||||||
|
|
|
★ |
|
|
|
❘■➚ |
|
✍✂✠✂❿❿✂ |
|
✠✟☛✡✞✗✑☛✆✆✑✔✞✡✠☞☛✌☞✍✂✖ |
|
|
✑✏✔ |
||||||||
|
✧✾➭ |
✑✔✞✡✠☞☛✌☞✞✗✠❆ |
|
|
|
|
|
|
|
|
✁✗ |
|
|
✩■ |
|
|
||||||
|
|
❿✂ |
|
|
|
|
|
|
|
❼❅ |
|
|
|
|
❳✂▲ |
|||||||
|
|
|
|
|
❢●☎ ✯✌ |
|
|
|
|
|
|
|
|
✑✏✔ |
|
|
|
★ |
||||
|
|
|
|
|
|
❿✂ |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
❙✌✒✎✍✑✏✄✖❳❞☎✱❪✝❜❳❜❯✼❘☛✸❣✩✝❵❣❱✼✩☎✖❨★✰✖❣✩✝❵✷✙☎✶✝✛☛✫✟☞✁✛❘★✝✠☎✠❲✬ |
|
|
|
|
|
|
|
|
||||||||||||||
❿✂ |
|
✟✞✡✠☛ |
|
❆ |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
||
❿✂ |
|
❿✂ |
✟✞✡✠☞☛✍✌✏✎✒✑✔✓ |
|
★ |
❿✂ |
|
|
|
✆ |
|
❖◆☞✓✒✕✔✆✖ |
||||||||||
|
❊✌ ❘✾ |
|
|
|
✁✆✥ |
|
|
|
|
|
|
|
|
|||||||||
|
✦✶✠ |
|
✟✳✏✎✑ |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
✒✓✄✕✔✗✖✘✄✝✙✚✞✏✛✜✌✣✢✥✤✦✛✜✧✚★✑✤✦✩✥✤✦✛✜✙✫✪ |
|
|
|
|
|
|
|||||||||||
❷❉➔✞➇➐⑩➍ ✹ |
|
|
✮ |
|
❈✒❈❉❊✮ |
|
|
|
|
|
|
|
|
|
|||
✭✬✯✮➈ ✛✜✆✰✄⑥ |
|
✏✛✹✡✰✺✍✻✼✢✥✤✽✛✧✚★✑✤✦✩✥✤✽✛✕✙✷✪✾✱✕✶ |
|
|
|
|
|
||||||||||
✩✥★☎✙✷●❂✄✜✒◗✲✿✭✎☎✄✝✞✍✎❁❀❂★✝✙✘✡☞✌❘✛✕✧✚✄ |
|
|
|
|
|
|
|
|
|
||||||||
✩ ✙✫●✗✄✜❍■✛✄✜✤✍✸✮ ✞✍✎❁❀❂★❁✌✺❃✻❄✱❅✢❇❆✮ ☎✹✲✿❏✎☎✄✫✞✍❑✥★❁▲❂✺❃✻✽✳▼ ☎✹■✿✭✎☎✄✝✞✼✶✫✄✝✞✹✺❃✻✽✳✚◆❖❆❈✳✵✳ |
|
|
|
||||||||||||||
|
✮❁✮✌❘✄✕✶✝✶◗✱■☎✹✲✿❚★✮❁✮✌❘✄✕✶✝✶ |
|
|
|
|
|
|
|
|
|
|||||||
✴❃✆✟✞❙★☎✙✚✞✍✴✍✛✜✆❖✱■✩✮✄✝✤✏✸❯✥✌✕✛✕✧✚✄☎✺❱★✝✳✽✡☞✌❘✛✜✧✚✄✕✻ |
|
|
|
|
|
|
|||||||||||
|
☎✹■✿❏★✌❘✄✕✶✝✶❈✱✲✮❁✮ ☎✹ |
|
✥★✑✙✷●✗✄✕❍■✛✟✳ |
|
|
|
|
|
|
|
|
||||||
|
|
✲✿❏❨❩✴❃✆✹ ✪✳❬ ☎✹■✿❏❨❭☎❳■✿❏✎☎✄✝✞✍✆❲✄✝❳☞✞✹✺▼✳✮❁✮✌❘✄✜✶✜✶❪ ☎✹■✿❏✧✚★✑✶✝✄❬✙✫★☎✙✷●✗✄✜✤❫✴❃✆✰✄✜✶✫✴✍❴❁✄✕✻ |
|||||||||||||||
✩✥★☎✙✷●❂✄✜❍■✛✄✜✤✍✸✶✝✄✫✞✍✎☎❀❂★❁✌✮ ✮✡☞✌❘✛✜✧✚✄☎✸✦✡☞✌❘✛✕✧✚✄✎☎✄✝✞✍❑❈★☎▲✗✺✍✻✳ ✲✿❏✎✑✄✫✞✼✶✝✄✫✞✹✺✍✻✝✳✹ |
|
|
|
|
|||||||||||||
|
|
|
|
✡✗✌✕✛✕✧✚✄☎✸✼✶✫✄✝✎☎✄✝✞✼✶✫✄✝✞✹✺❃✻❬✻ |
|
|
|
|
|
|
|
|
|||||
|
|
|
|
✡☞✌❘✛✜✧✚✄☎✸❑❈★✎☎✄✝✞✍❑❈★☎▲✗✺✍ |
✼✻ |
|
|
|
|
|
|
|
|
||||
|
☎✹■✿❚✶✫✄✝✞✍✮✺❃✢❇❆✎❁❀❂★☎✌✥✒◗❉❊✮ |
|
|
|
|
|
|
|
|
|
|
||||||
|
|
|
✴❃✆❵✶✫✄✝✌✕✞✹✺❱ |
|
|
|
|
|
|
|
|
|
|
|
|||
➇➍★✑✙✚✞✍✴✍✛✜✆❖✱❅❍☎❛✵❛✲ |
|
|
|
|
|
|
|
|
|
|
|
|
|||||
✎☎ |
|
|
✎✑✄✫✞✍✎❁❀✟★❁✌✺❃✻✮ |
|
|
|
|
|
|
|
|
|
|
||||
➇➍✎☎ |
✬◆❖❆❈✳❄✳⑥✮✬✎☎❀❂★❁✌✢❇❆✥✒◗❉❊✮ |
|
|
|
|
|
|
|
|
|
|||||||
✎☎ |
✲✿❏✆✰✄✫❳☞✞✏✤✦✄✫❳✰✺ |
✢✤✽✛✕✧✚★☎✤❱✩✥✤✽✛✕✙✷✪☞✻ |
|
|
|
|
|
||||||||||
☎✹❝✿❝✶✝✄✫✞✡☞✌❘✛✕✧✚✄❁✸✼✶✝✄✝✞✹✺✎✑✄✫✞✼✶✝✄✫✞✹✺✍✻✼✻ |
|
|
|
|
|
|
|
|
|||||||||
|
|
|
|
|
✡☞✌❘✛✕✧✚✄☎✸✎☎✄✫✞❑✥★❁▲❂✺❑★❁▲❂✺❃❬✻✍ ✥ |
|
|
|
|
|
|
|
|||||
➁➁➁ |
|
|
✴❃✆✰✶✝✄✫✌✕✞✹✺❱✎☎ |
|
|
|
|
|
|
|
|
|
|
||||
|
|
|
|
❊⑤✢✤✣❴❥✺✧✐⑤❢❞✈❞✢✼❡✏❢✈⑤☎❝☞❧ ✂❦✜✍♥❴❝❮✇✞⑤❢②✎♠❴❡✟❦❳❦✈⑤❢♥✉ |
✟✞✡✠☞☛✍✌✏✎✒✑✔✓✆✆☎✞✡☛✑✔✞✗✠☛ |
||||||||||||
❘■✂ |
|
|
|
|
|
✕✝✙✐❣❂●✉☞✍✌✈✙✾❚✯✌ |
✧✾❃✟✂ ❿✂ |
|
|||||||||
✧✾ ✠✂❿ |
|
|
✡❤❣✔ ✁ |
|
|
|
✚❘❛✧✾✴✯✌ |
➆❅ |
❈✂ |
★ |
✧✗ |
|
☎✞✡☛✑✔✞✗✠☛✓■★✧✾✴ |
||||
✁❿ |
|
|
|
✒✔✆✖❨✓ |
✙✾❚ |
✄❅✻✧✗ |
❿✂ |
|
✌●✔➣ |
|
|
|
|
|
|||
❥☛❦✑✦ |
|
|
|
|
✓❏ |
✧✾❪✙✾❊ |
✙✾ |
|
|
|
|
❂✾✖ |
✯✌ |
❊✂ |
|||
|
|
|
|
✧✾✴ |
|
|
✒✕✔✆✖✡➚✏✟✩✾❉ |
|
|
|
|||||||
|
|
|
|
|
|
|
|
|
◗ |
|
✧✾✴ |
|
|
|
|
|
|
❿✂✑ |
❩❅✠✒✔✖✡✼ ❱ |
❢●❍✁■➚ |
|
|
|
|
|
✁✗✧✾❊ |
✑ |
||||||
|
|
|
✙✾✴1 |
1 |
2 |
3 |
4 |
5 |
6 |
7 |
8 |
9 |
❉ |
||
|
|
|
10 11 12 13 |
||||||||||||
|
cls = 32 |
|
|
|
|
|
✙✾ |
|
|
|
|
||||
|
for I = 1, N |
|
2 |
|
|
|
|
|
|
|
|
|
|
|
|
|
for ✂❿J = 1, N |
|
|
|
|
|
|
|
|
|
|
|
|||
|
|
|
|
|
|
|
|
|
|
|
|
|
|||
|
|
3 |
|
|
|
|
|
|
|
|
|
|
|
||
✁❍ |
◆✙✾❁I[0]✩✾❉= J |
|
4 |
|
|
|
|
|
|
✑ |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
||||
|
I[1] = I |
|
5 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
6 |
|
|
|
|
|
|
|
|
|
|
|
|
prod[I] = 52 |
|
7 |
|
|
|
|
|
|
|
|
|
|
|
|
|
prod[J] = 8 |
|
|
|
|
|
|
|
|
|
|
|
|
||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
||
|
min |
= [1 , 1] |
8 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|||
|
max |
= [11, 7] |
9 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
11 10 |
|
|
|
|
|
|
|
|
|
|
|
✧✾ ✁❮ |
❂✾✂❿ |
|
|
|
|
curr = [I=5, J=3] |
Ex. 1 |
|
address✁=❍224 |
✧✾❩ |
|
|
||
offset = 224%32 = 0 |
|
|
inc = 32/8 = 4 |
|
|
prov = [I=5, J=7] |
|
|
done? = 7 <= 7 |
|
|
curr = prov |
|
|
new address ❿✂= 256 |
✑ |
|
curr = [I=5, J=7] |
Ex. 2 |
|
address = 256 |
|
|
|
|
|
offset = 256%32 = 0 inc = 32/8 = 4 prov = [I=5, J=11] done? = 11 <= 7 prov = [I=6,J=1] done?
done? 5 <= 7 curr = prov
new address = 292
❮
✍✂▲✙✾
❙✌✒✂ |
✄✖❳❞✏☎✹✻✖❳❞✝❵✷✛☞★✼✭ |
|
❊⑤✢✤✣❴❥❅ |
✁✧✿❝✽✫✙❦✂❦✡✜ |
❳❦✈❝✣✢✐⑤❢❞✈❞✩✖✫❴❦✡②★♠✱❡❢❝✆❞ |
|
|
|||||||||
|
|
✔✗✖✶✢✧✖✙★✰✖☛✯✷✫✟☞✁ |
✛✩★✝✠☎❭❲✬ |
|
✍✂➭ ✩■ |
❮ |
✺❋❉ |
|
||||||||
☎ |
|
|
✁✗ |
|
❲✦✝❵✷✍✼✩✟✏ |
|
❍ |
✍✂❆ |
❏ |
✁ |
||||||
|
❿✂ |
✁❿ |
✍✂ ❿✂❍❳✂❉ |
|
|
|
|
✩■ |
✖ |
|
|
|
||||
|
|
✩■ |
✯✌ |
✧✾❰ |
|
|
|
|
||||||||
❙✌✒☎✄ |
|
✍✂❰ |
|
|
|
|
|
|
✁✗ |
|
▲◆ |
|
||||
☎♦✼✩❜▲■☎✞✝❜❵✷ |
|
|
❈ |
✧✾➭ |
|
|
|
✩■ |
|
|
|
|||||
❁✄✁❉❚✿ |
✿✂ |
❛❘ |
|
|
|
|
|
|
|
|
✍✂☛✂❿❮ |
|
✄✂✴✁ ✧✾✁✴ |
|||
❉ |
❿✂ |
|
❍✏✂✔✄✁❍ |
✙✾❉ |
|
|
✁✗ ★ |
✿✂ |
|
|
||||||
|
|
|
|
|
|
|
|
|
|
|
✆ |
|
|
|
|
|
⑥ |
|
✧✤✧ |
|
|
|
✭★✑✔✞✏✄✝✌❁✻ |
|
|
|
|
|
✶✷✞✏★✑✙✷✪❘✆✰✄✫❳☞✞✏✤✦✄✫❳✰✺✍✒✓✄✕✔✗✖❇✄✜✙✚✞✏✛✜✌❬★✑✔☞✳ ✫✌❘✤❫✴❃✁☎✄ |
|
|
|||||||||
|
|
|
✞✏●☞✴✏✶✝✤❫✴❃✁☎✄✂✞✍✌❘✄✝✝✌✕✸✲★✑✔✱ ☎✹✜✄▼✡☞✞✍✌ |
|
|
|
|
||||
|
✏●☞✴✏✶✫ |
✝✹❅✿✭✞✍✌✕❈❝✞✏●☞✴✶☎✹✲✿❏✎✑✄✫✞✏✤✽✴❱✁✑✄❁✺✍✻ |
|
|
|||||||
|
|
|
✺☞✽✄✝✆❖✱❝❨❭✴❱❱✆❲✞✗✂✱✲✴✄✂✼✠☎❏✞✏●✗✴✍✶✝✤✽✄✆✹✥✁✂❬✌✡✝☛✝✂✻⑥ |
|
|
|
|||||
✴❃✆✟✞✘❨❩ |
❃✆❲✤✦✄✫✆❖✱❝✞✏●☞✴✏✶✫✤✦✄✫✆❖✱✲✞✏●☞✴✏✶★✹■✿❚✽★✶✷✞ |
|
|||||||||
|
✴✏✔✂✞✏●✏✶✝✤❫✴❃✁✰✼✗✱✲★✔✞✏✄✫✌❘✤✽✴❱✁✑✄❅✿✭✎☎✄✝✞✏✤❫✴❃✁☎✄☎✺❃✻✰✼✦✻❲✙✚❀✗✕✌✴✰✼☞✱✲★✑✔☞✞✏✄✫✕✸✙✚❀❂✌✜✌✴✰✴✼ |
|
|
||||||||
★✑✔✞✏✄✝✌❘✤ |
|
✔ ✪✳❬❨❭✴❃✆❲✦✄✫✆❵✻✵✺❱★ |
|
|
|
||||||
✔☞✛✜✌✺✴❃✆❲✞✗✴✄✂❬✴✆☎❏❨❩✴❃❲✤✦✄✫✆✼✴✞✝✟✝✂✻ |
|
|
|
|
|
||||||
|
✄✜✤✦✶✝✄✵✔☞✛✜✌✙✚❀❂✴✰✼✱✲❨❭✴❃✆❞✸✙✚❀❂✜✌✞✏✄✝✌✕✤✦✄✫✆✴✰✼ |
|
|
|
|
|
|||||
|
➁✧✹✌❘✄✜★☎✪ |
|
|
|
|
|
|
|
|||
✴✏✔✂✞✏●☞✴✏✶✝✤✽✌❘✤✽✄✝✆✱✲★✑✔✄✝☞✭✌✥✱✲✞✏●✗✴✍✶✝✤✽✄✝✆✌ ✍☎❚★✔✞✏✄✫✌⑥ |
|
|
|||||||||
➁✆✰✛✝✌✕❨★☎✤❫✴✍❴❁✄☎✺✦❨❭✴❃✆✖✳▼❨❭★❁❳❂✻ |
|
|
|
|
|
||||||
➁ |
✤✽★✶✷✞✹✺✍✻✌✝✟✝ |
❊⑤✢✤✣❴❥❑ ✁✧✿❝✽✫❦✴❝✘✽✫✞⑤☞♥★✣✴❧❃❦✡♠❂♣❴⑤✆❦✡❡♥✏✎✪❝✽✬↔❝✣❧✈❝☞❂♥☞❝✯❡✤✣✉♥✴❧✻⑤❦✞♣✱② |
|||||||||
|
❿✂ |
|
|
||||||||
★ |
|
|
|
|
✍✂ |
✁★ |
❍ |
✧✾❉ |
|
❿✂ |
|
▲✿ |
❿✂ |
|
|
|
|
|
|
✙✾❉ |
|
||
|
|
|
✁✗ |
|
|
❍ |
|
||||
❉❁ |
✍✂❿ |
|
|
❩❋✆✔ |
✙✾✴▲◆ |
|
|
|
✙✾✴✍✂ |
✍✂ ✏✟ |
|
|
|
|
★ |
|
|
|
|||||
❿✂❉❁✄✁❉❁ ❍✎✔✏ |
|
|
|
|
|
✠✂❿ |
|||||
✷❮❂◆▼✞❇❊✺③❭❪❇❊❏❴❫✯❋✁ ✏✂✂✒✑❆❇❉▼✱❋✔✓❿✂ |
|
|
|
|
|
||||||
|
✡✕☛✪❯✗✖✘✑❮❂◆✺✻✸✼❏➭❋✴❀✈❋✔❄✍❋✴✽✿❏✭❋✁ ✸✙✑❆❇❉▼✱❋✔✓❿✂ |
|
|
||||||||
❅❛❋✔❄✚✑➭❋✔❀❃❋✔❄✍❋✴✽✿❏✭❋ |
✔✓✄✂☎✂❿ |
|
|
|
|
||||||
|
|
|
★ |
|
|
|
|
✜✛✿ |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
❍ |
✁✗ |
✗✆ |
✍✂ |
★ |
|
|
|
|
|
|
|
|
|
★ |
|
❅❛❋✔❄✁❆❄✱❄✍❇✕✽ |
✂✱✸✼❏✁ ✸✄✂☎✂✒✑❆❇❉▼✱❋✆✓✂❿ |
|
|
|
|
|
|
|
|
|||||||
❅❛❋✔❄✁❆❄✱❄✍❇✕❭✖❇✗❏✱❫✯❋✽ |
✯✑➨ |
|
✗✑❆❇❊▼✞❋✔✓✂❿ |
|
|
❇❏ |
|
★ |
||||||||
★ ✌✿ |
★ |
✲◗ ✁③ |
★ |
|
|
|
✌✯ |
|
❃ |
✆ |
✁③ |
|
✂❿ |
|||
|
|
|
|
|
|
|
|
|
✧✾❊ |
|
||||||
✂☎✄✝✆✌✭✒✟✞✍✌☎☛✍✌✡✠❈✒☞☛✍✒☎☛❋✮✍✌✏✎✥✙✒✑✚✙✚★✔✓✤✙✚✿ |
|
|
|
|
✤✣✭✒☎☛✤✒✭✮☞✥✍✒✏✎✦☛✧✌✦✔✠★✜✘✩❍✕✝✜✘✩✘✩ |
|
||||||||||
✓☞✕✖✠✴✏✲✔✗✭✲❋✮✠✘✌ |
|
✙✓☞✕✖✠✴✏✲✔✚ |
✍✌✘✛✘✜✧✿✧✩✢✚ |
|
||||||||||||
✚■✻✏✮✧✮✏✭✮✤✣✭☎☛✚☛✒☎✲✡✠✘✌✦✔✠❀✘✜✧✿✘✩✘✩☎★✫✪✦☛✎✧☛❈✻✎❍✮✭✻■✻✏✮✧✮✏✒✭✮✬✄✭✚☞☛✍✒✥☛✍✌✮✌✜✦✔✠✜✦✦✦■✻✏✮✧✮✏✘✛✘✜✿✩✧❀✧✛ |
|
|
||||||||||||||
✞✪✦☛✎✞✥✣✂ |
✯✄✏✒✒☎✱✰☛✍✒✏✎✭✌☞☛✳★✍✦✧✛✧✦✧✩✮✌ |
|
|
|
|
|
|
|
|
|
|
|
||||
✣✤✍✒☎☛✧✲✍✛✧✦❍✕✝✩✚✛✘✩✘✛✘✩✦☎★✍✦✤✦ |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
||
✂✦✥✦✚✦ |
✯✄✏✒✝☛✍✌✮✌✳✦✔✠✿✘✜✧✩✷✪✦☛✎✍✒☎☛✭❉✲☛✭✴✖✕✘✣✸✪✦☛✎✍✒☎☛✧✲✍✒☎☛✍✒✏✎✯✄✏✒✢☛✍✌☞☛✮✌✳✦✔✠✖✕✯✦✧✦✧✩✧✦❍✕ |
|||||||||||||||
✲☛✭✴✖✕✘✣✴✳✧✒✦✵✏✲✶✪✦☛ |
||||||||||||||||
|
✻✠☞☛✍✌✏✎✍✑✍✒✤✙✤✛✽✷❆✕✯✛✘✛✽❁❃❂✔✠☞☛✍✌✏✎✍✑✍✒✤✙✤✛✽✷❆✕✯✛✩✘✛✘✩ |
|
|
|
|
|||||||||||
|
|
✧✦☎★✍✛✯✺✭✛✔✠★✩✧✦✩❍✕▼✞ |
✼✻✤✦☎✗✤✦✚✦ |
|
|
|
|
|
|
|
||||||
|
✿✘✜✘✩✘✍✒☎☛✧✲✍✒☎☛✍✒✏✎❍✕✠✜✛✿☎★✤✣ |
✍❉❋✕✘✗✔✩✥✦✚✦✧✩ |
|
|
|
|
||||||||||
|
|
|
|
|
|
|
|
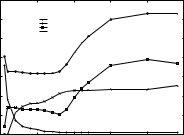
Blocked Transpose K =1, L = 4, N = 9 |
|
|
|
|
||||
|
✛✘✜✘✛✘✜✯✺✘✜✜✿ |
|
0.7 |
❊❉❋✤✛☎ |
|
|
❀✧✦✧✦✧✦✢✦☎★✝✦✘✛✘✜✥✦✶✕ |
|
|
|||||||
|
|
|
|
|
|
|
||||||||||
|
|
0.6 |
✘❇❈✕✸✷Miss✱✩☎✗Rate ✤✦●❂☎✗✟✛✘✜✘✛✘✤✦✚✜✥✦ |
|
|
|
|
|||||||||
|
|
|
|
|
|
|
Instrinsic Rate |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
||
|
✹★✍✦✧✛❍✕✘✺❄✛✧✩✔✩✧✛✚✣✡✓✖✻✠✢✕✘★✡★✩✘✩✚✣✡✓✖✻✸✷●❂☎❇❈✕✘✗✼✻✚★✧✗✤✦✥✦✦✚✦✥✛✧✦☎★✍✛✢✦ |
|
|
|||||||||||||
|
|
|
|
|
0.5 |
|
|
|
|
|
|
|
|
✴♠❂❧☞✣❝✶♥✔⑤❢❞✘✶❦✻⑤❢✴✣ |
|
|
|
|
|
|
|
0.4 |
|
|
|
|
|
|
|
|
|
||
|
|
|
|
|
Rate |
|
|
|
|
|
|
|
|
|
||
|
|
|
|
|
0.3 |
|
|
|
|
|
|
|
|
|
||
|
|
|
✢✤✣❊⑤❴❥◆✧✜✂❦✍♥❴❝❮✇✙❦✈❦❳❦✈⑤❵❦♥❢❞☞❞✿❦✡✏✯ ✍❦❃❦❳❦✻❝☞ |
|
|
|||||||||||
|
|
|
|
|
0.2 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
0.1 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
0 |
0 |
50 |
|
100 |
|
150 |
200 |
250 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|||||||
Block Size
✢✤✣❊⑤❴❥✧✪✩✖✫❴❦✡②★♠✱❡❢❝✚❧❂♣❃❦✡♠❈♥✿❱✿✟✬☞✢✐⑤❢❞✈❞➓❦✎❳❦✈❝✣✜ |
✙❦✻❞ |
✟ |
✑➭❋✂✞❑✯✺❼▼☎✂ ❍ |
|
✂❿ |
|
|
|
|
|
|
|
|
|
|
✏✭ |
✍✂✴ |
|
|
|
|
|
|
||||||
|
9e+06 |
|
|
|
|
|
|
✏✭ |
|
|
|
||
|
|
|
8e+06✂❿ |
Mat. Mul. L 6 N 7 K 4 |
|
|
|
|
◆ |
|
|
||
|
|
|
Mat. Mul. L 8 N 7 K 4 |
|
|
|
|
|
|
||||
|
|
|
Mat. Mul. L 8 N 4 K✁4 |
|
|
|
|
|
|
||||
|
|
|
|
Jacobi |
L 7 N 8 K 1 |
|
|
|
|
|
|
|
|
✍❱✒✠✑ |
|
|
Jacobi |
L 7 N 8✏✔K 4 |
|
|
|
|
|
|
|
||
|
7e+06 |
|
|
|
|
|
|
|
|
|
|
||
Second |
6e+06 |
|
|
|
|
|
|
|
|
|
|
||
|
|
|
|
|
|
|
|
|
|
|
|||
✝✬❛❖✟✁✼✩☎✞✝✛☛❨❬✖✙★❭✢✧✛✩★✴✬✼✩☛✸✷5e+06 |
|
|
|
|
|
|
|
||||||
|
|
Per |
|
|
|
|
|
|
|
|
|
|
|
|
|
References |
4e+06 |
|
|
|
|
|
|
|
|
|
|
|
|
3e+06 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
2e+06 |
|
|
|
|
|
|
|
|
|
|
|
|
|
1e+06 |
|
|
|
|
|
|
|
|
|
|
|
|
|
0 |
0 |
10 |
20 |
30 |
40 |
50 |
60 |
70 |
80 |
|
|
|
|
|
||||||||||
|
|
|
|
|
|
|
|
Block Size |
|
|
|
|
|
✿✌✗✆❪✿✜✛➲ |
|
▲✾✁③❊⑤✢✤✣❴❥✦✥✯◗☎✧✪❝✎✽✬↔❝✣❧✈❝☞❂♥☞❝➨✇✞⑤❢②✎♠❴❡✟❦✔✆ ❳❦✻⑤❢♥✉➓❦✎❳❦❃✟✈❝➐♥✬✴❧✳❦✢❳❦✞❧✻⑤✫❿✂❄♠✢❴❡✈⑤❢♠✱❡❢⑤❦ ♣❦♥❳❦✻⑤❢♥✉❮ ✁■➚ |
|
|
||||||||||||||||
|
|
✍✂✥ |
✧✾❉ |
|
❘■✂ |
✁■➚ |
✧✾❉ |
❿✂ ❍ |
❿✂ |
|
|
|
❿ |
✙✾✴ |
|
★ |
||||
❉ |
|
|
|
|
✏❋✴ |
❆❁❊✗☞ |
|
|
|
|
|
|
|
|
||||||
✁❮ |
|
|
|
|
|
|
|
✧✾❉ |
|
|
|
|
|
|
|
|
|
|||
|
|
|
|
✍✂❉ |
|
|
|
|
|
|
❿✂ |
|
|
|
|
|
❅❼ |
✍✂ |
||
❪❣❁❱●✛❮ |
|
|
|
✁✾❆ |
|
|
|
★ ✞ |
❣◗✕☛ |
|
✆❣✖✕✠ ❣◆ |
|||||||||
|
|
✂❂✾❉❱❋❉ |
✍✿▲❆✿ |
|
|
|
✁✾ |
|
|
|||||||||||
|
❘✂▲✴❋ |
|
|
✂✁✄✁✎ |
|
❍◆☞ |
|
|
|
❮✎ |
|
☎✂❿ |
|
|
||||||
|
|
|
|
|
★ |
|
|
✿❂❁ |
|
|
|
|
|
|
|
|
||||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
❘✂✴ |
▲➚ |
|
|
|
✝✁❍✖✴❜✁■➚ |
✭❛❤✟✡✝❵✷❪ |
|
✏✭✿❱✿➭✍✂✴ |
|
|
|
|
|
|||
✂❿✍❱✒❚❙❯✛❘✬✮✭✝✠✟✖❡♦☎✶✝✬✲✖❆❜✙✖✟✷✙☎✶✝✛☛✛❀✢✤✝✠✟✂❿✖✼ |
|
✌✯ |
▲✾❨ |
|
|
|
|
|
|||||||||||
❉ |
0.025 |
✁★ |
Matrix Multiply Associativity = 4, Lines Size = 64, Lines = 128 |
❅✼ |
✁★ |
0.18 |
|
|
Matrix Multiply With Different Cache Architectures |
✌✯ |
|
▼✿✓✿ |
|||||||
|
|
|
|
|
|
|
|
|
|
|
|
||||||||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
||||||
|
|
|
|
|
|
|
0.16 |
|
|
|
|
|
|
|
|
||||
|
|
|
|
|
|
|
|
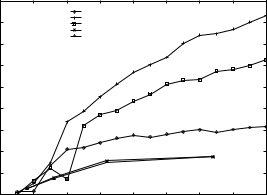
K = 1, L = 6, N = 11 Miss Rate |
|
|
|
|
|
||||||
|
0.02 |
|
|
|
|
|
|
✡✁✌◗K =❭●4,✉L = 6, ✂❿N = 9 Miss Rate |
|
|
|
|
|
||||||
|
|
|
|
|
✾✴ |
|
|
☞❱❁✠ |
0.14 |
K = 4, L = 3, N = 12 Miss Rate |
|
✁■ |
|
|
|
|
|
||
|
|
|
|
|
|
|
0.12 |
|
|
|
|
|
|
|
|
|
|||
|
|
|
✂❿ |
|
✁ |
|
|
|
✁◆ |
|
|
|
|
|
|
|
|
|
|
|
0.015 |
|
N = 293 Miss Rate |
|
|
|
|
|
|
|
|
|
|
|
|
|
|||
|
Miss Rate |
|
N = 300 Miss Rate |
|
|
Miss Rate |
0.1 |
|
|
|
|
|
|
|
|
|
|||
|
|
|
|
|
|
|
0.08 |
|
|
|
|
|
|
|
|
|
|
||
|
0.01 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
0.06 |
|
|
|
|
|
|
|
|
|
|
|
0.005 |
|
|
|
|
|
|
|
0.04 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
0.02 |
|
|
|
|
|
|
|
|
|
|
|
0 |
20 |
40 |
60 |
80 |
100 |
|
120 |
0 |
20 |
40 |
60 |
80 |
100 |
120 |
140 |
160 |
180 |
|
|
|
|
|
||||||||||||||||
|
|
|
|
Block Size |
|
|
|
|
|
|
|
|
|
Block Size |
|
|
|
|
|
|
✿✌ ✓❏ |
|
❊⑤✢✤✣❴❥✦✥★✥★✧✳❦✢ ❳❦✶❧✈⑤✫❄♠✢ ✱❡✈⑤❢♠❴❡❢⑤❦ ✆❦♥❳❦✻⑤❢♥✉➐♥✬✴❧✉❦✍❧✈⑤❢♥✴♠✱❞✰✉❤✵ |
✪❦✜ ✍♥✞♣❴❝❧✞♥✞♣❴⑤✈❝❦✣♥♦❦✶♠❂❧✈❝☞❞ |
|||||
|
✯✌ ✿❇❁✥✿✁③☞ |
✙✾❊ |
❉ |
★ |
✁✾✔ |
✁✿✡✍✌✛ ➘ ❮ |
|||
▲❏✭✴✥ ✖✕✧✫☎✒✤✞✡✓✵ ✼✓✔✷★✓ |
✼✓★✓✧✗✡✠✂ |
✼✓✔✷★✓✧✗ |
|
|
|
|
|||
✰ |
✲✭✴✏☛✂ |
✧✗✔✷❃✣✡✓✄✂ |
|
|
|
|
|
||
✵ |
|
✄✂ |
|
|
|
|
|
||
✱ |
|
✻✼✙✶✻✸✷✠✎✹✻✧✻✘❇☎✂✔✷★✓✺ |
|
|
|
|
|||
✺ |
✲✭✴✏☛✤❂✧❂✚✙✶✕✸✷✧✻✼✙✶✕✸✷✥✙✠✎✹❂✧❂☎❇☎✂✔✷★✓✺ |
|
|
|
|
||||
❅❑ |
|
✒✏✎✍❏✞✡✓✖✻✸✷●❂☎✗✧✙✧✞✡✓✖✻✘❇❈✕✸✷●❂☎✗✧❇✧✣✡✓✖✻✸✷●❂☎✗✍❇✧✣✡✓❆✻✸✷●❂☎❇■✕✘✗✧❇✂✓✜❂✽✷✘✻✘✗✧❇✂ ✻✘❇❈✕✯✗ |
|||||||
◆✯◗✒✏✎✍❏✒✏✎✍❏✒✏✎✍❏ |
|
|
|
|
|
|
|||
✥★✥✸✫✸✷✸ |
|
|
|
❊⑤✢✤✣❴❥✦✥✵☎✧✪⑤❢❡❢❝☞✿☞✧❦✈❝☞❂♥☞⑤❢❡✜ |
|
|
|||
|
|
|
|
|
|
|
|||
Tiled Stencil Computation
|
0.24 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
L 6 N 7 K 1 |
|
|
|
|
|
|
|
|
|
L 5 N 7 K 2 |
|
|
0.22 |
|
|
|
|
|
|
L 4 N 4 K 4 |
|
|
0.2 |
|
|
|
|
|
|
|
|
|
0.18 |
|
|
|
|
|
|
|
|
Miss Rate |
0.16 |
|
|
|
|
|
|
|
|
0.14 |
|
|
|
|
|
|
|
|
|
|
0.12 |
|
|
|
|
|
|
|
|
|
0.1 |
|
|
|
|
|
|
|
|
|
0.08 |
|
|
|
|
|
|
|
|
|
0.06 |
|
|
|
|
|
|
|
|
|
0 |
20 |
40 |
60 |
80 |
100 |
120 |
140 |
160 |
|
|
|
|
|
Tile Size |
|
|
|
|
✿✌ |
✢❊⑤✤✣❴❥✥✰0.65✧✔✎0.7➓❝☞❞✶♠❴❡✈❞❦✬➐♥✴❧✔☎➓⑤❢❡❢❝☞✙❦✈❝☞❂♥☞⑤❢❡❉❤➘❝✣❧✻ ✦✬➐♥✴❧✁➚❦✍❧✈⑤❢♥✴♠✱❞✙✜✂❦✍♥✱❝✣✂✗❦✍❧✈❦✡②★❝✣❦✻❝✣❧✈❞ |
◆ |
|||||||
✁✾➭✿✙❈ |
0.55✲✿❛ |
✁❁ |
|
❍ |
|
|
|
||
|
|
|
|
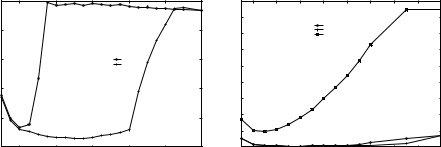
Tiled Transpose Simulation vs. Real |
|
|
|
||
|
Degradation |
0.6 |
Target |
|
|
|
|
|
|
|
Performanceor |
Simulation |
|
|
|
|
|
|
|
|
0.5 |
|
|
|
|
|
|
|
|
|
0.4 |
|
|
|
|
|
|
|
|
|
|
0.45 |
|
|
|
|
|
|
|
|
Simulated |
0.35 |
|
|
|
|
|
|
|
|
Normalized |
0.3 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
0.25 |
|
|
|
|
|
|
|
|
|
0.2 |
50 |
100 |
150 |
200 |
250 |
300 |
|
|
|
|
|
||||||
|
|
|
|
|
Tile Size |
|
|
|
|
☎✂ |
❭❪❂➚✽✿❏✭✺✼❑✞✸✻❂◆✽✂ ✂ |
❊⑤✢✤✣❴❥✘✱✥✴✧☞✂❊❝❀❧✄✬↔♥✴❧✻②③❦✡❂♥☞❝➘♥✟✬☞☎✪⑤❢❡❢❝✆✳❦✢❳❦✶❧✈⑤✫❖❧☎❃❦✡ |
❂✾✔ |
||
|
❊ |
❢✂✴ |
✠✂❿ |
✿ |
|
|
|
|
|
|
|
|
|
|
|
✡✆ ✈✌ |
❊ |
|
▲✿✡✜✛✁✌❃ |
|
|
|
|
✄✂❿❮✑ |
❮ |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
★ |
|
|
|||
❆❏✪✽✿❂✄✂✥✺✻❋✔❬✯✾➚❯❱❋✴✽✂ |
|
|
|
➧❮✠✡✒✭✎✏✎✏✠✡✝✣✁✞✠✙✄➳ |
|
|
❛✁✞✒✔✢➲✮✯✬✔✁✵✄☞✝✟ |
|||||||||
➹➘✬✭✠✖✁✞✩✭✘☞✬✭☛✞✄☞✎★✃➘☛✞✩✔✝✣✢ |
|
|
|
|||||||||||||
➹◆✠✡✌♣✬✭✒✔☛✞✝✣☛✞✓✱✑✟✠✡✎✡❒✠✟✯✠✙✝✣✝✔✕➚✁✵✫❉☛✞✄✆ |
☛✡✌☞❮✄✆✁✵✒✦✘☞✎✿✮❧✮✯➧➣➷✑✟✠✡✯✁✵✒✴✢❛✫✦✶❛➵❿✤✎✍✑✏✁✒✔✓✖✕✗✏✖✕❮✁✞✒✔✢✐✮❧✮✯➧➣➷✘✍✁✒✖✕✚✙✁✛✑✏✢✜▲➮✯➹➘✬✔✠ |
|
||||||||||||||
|
|
➸❺☛✵✄③✬✔✑✟✎★✬✔✠✡✝✟✲❆✑✟✒➲✑✟✰✳✲✔✝✟✠✡✰✳✠✡✒✍✘☞✑✟✒✔✓✳✘☞✬✭✠➳✲✴✁☎✄☞✎✏✠✙✤✣✔✠✡✌✙✘➣✘☞✬✔✠❍✲❉☛✱✎✏✑❢✘☞✑✟☛✱✒✥☛✞✄➨✲❉☛✞✝✣✑✟✌✙✶❪☛✞➸➚✘☞✬✔✠❈➮✤❉➮❍☛☎➩❴✠♣✄☞✒✔✰✳✠✡✒✍✘✉➮✑✣☛✞✒✹☛✞➸✗✘☞✬✔✠❍✎✏✑✟✰❈✩✔✝✣✁✵✘☞☛✵✄✉➮➓➹➘✬✔✑✟✎✯✃➘☛✵✄☞➬✐✃❧✁✵✎❧✎✏✩✔✲✔✲❉✥ |
||||||||||||||
✑✟✒✹✘☞✔✠❿✮✝✆✞✆ |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
||
✌✙☛✱✒✍✘☞✠✡✒✍✘➨✢✭☛✍✠✡✎➨✒✔☛✵✘➣✒✔✠✡✌✙✠✡✎✏✎☞✁✵✄☞✑✟✝❢✶❰✄☞✠❆❋✴❀❃❋✭❄✍❋✴✽✯❏▲❋✂ |
✏✄✆✁✞✒✔✎❃➸↔☛✞✄☞✰✹✁☎➷ |
|
||||||||||||||
✱➮✕ |
✛✧✦➮❮✫✭✩✭➷✈✤✭✁☎➸➆✁✞✬✗❒✝★➳➮✿✧✩★✩✔✌✆➬❉❒❧✁✞✒✔✢✪★➳➮✬✫ |
✭✦☞✑✟✠✱➮ |
|
|
||||||||||||
|
|
|
|
|
|
|
|
|
❛➮➘✕◆✁✉✃❧✗✰✆✔✱✳✲✖✴✶✵✸✷✺✹✼✻✁✽✖✾✔✽❀✿✞❁✔✷❂✱➨✩✭✘☞☛✞✰✹✁✵✘☞✑✟✌❚✲✭✄☞☛✞✓✞✄✆✁✵✰✏✶✳✌✙☛✱✰✳✲✔✩▲☞✠✙✄☞✎✡➮✂➱✻✒❼✔✲✄❁✁❃✢❄✸❅✄❆✖✷➶ |
|
||||||
|
✘☞✑✟☛✱✒✔✎➘➸↔☛✞✄➣➩✦✑❢✄✏✘☞✩✴✁✵✝❉✰✳✠✡✰✳☛✞✝✟☛✍✌✉✁✞✝✟❢✘✈✶✍➮➨➱✈✒➻❛➶❏■ ❑✰▲✹▼✱✳✷✻❑✰✤❂❆✶❁✁❃❆◆❅✑❅▲✷❖✵✸✮➮▲➹➘✎✏✠✡✒✭✓✭➮✯✮✯☛✱✰✳➽ ❑❁✢❄◗❄◗✱✺✲✖✴❙❘❏❁✔✲▼✴✔❆☛❁✗✴✭✑✣✝✟✠✙✄➘☛✱✲✭✘☞✑✟✰✳✑✟➴✉✁☎✘☞✑✣☛✞✒✔✎✯➸↔☛✞✄❧✑✟✰✳✲▲✄☞☛✍❚❁✢✲❉❱❯❲❅✶❁✔✷❂✱✳✲▼✴ |
|||||||||||||||
|
➶✔✲✗✵❇✲❈✰✆ |
|
❉✍✁✙✁✍✗❊✖✍✁✓✢❋✭❒✗ ❀✕✗✍✑✓✢✍▲➮ |
|
|
|
|
|
|
|
||||||
✦➮★✤❊➮▲✮➘✁☎✄✏✄✉❒✒ ●✩✐➮✖❍❰✌✚✩ |
|
✛ |
|
|
|
|
|
|
|
|
|
|||||
|
➽✻❇❄➳ |
|
➽❁✔✲❩❨❇❳★✑✟✒✔✝✟✠✙✶✍❒▲✁✞✒✔✢✖✮✌✙✘☞☛✱✫❉✠✙✄▲➮ |
|
|
|
|
|
|
|
||||||
▲❿➹❍➮✜ ▼✡✴✁✵✬✭✄☞✑✟✒✔✓✞✠✙✄✉➮❭✦➨✩✭✘☞☛✱✰✹✁☎✘☞✑✟✌➨✮➘✞✌✆✬✔✠➨ |
|
|
|
|
|
|
||||||||||
|
➱✈✒✯✮❧✗✰♣ |
|
|
❰✶✵❫❪➻✵➶➚➻✻✁✽✑✽✁❴✔❳❵❘❑✰❬✕✚✍✁✍✔❋✆✘❛❪✷❜❁ |
❝✕✗✔✍✁✜✦➮ |
❫❍❰✠✡✰✳☛✵✹✮➘✁✵✌♣✬✭✠❰✁✵✒✴✢✞✄✏✶ |
||||||||||
✭➮❋✸★➳➮❭☞❿✁✵✒✔✒✭☛✱✒◆❒✛➮✂ |
|
❞✩✐➮❭☞ |
|
|
✍✘✏✄✆✁ |
|||||||||||
|
❚✁✞✒✔✁✞✓✱✠✙✰✳✠✡✒✍✘❪✫✍✶❍ ❡☞❍✝✟☛✫✔✁✝❿❿✁✞✝✟✝✟✑✣➩✞✁✞✒✗➮❩✤➹◆✄✆✁✵✒✔✎❃➸❺☛✵✄☞✰✹✁✵✘☞✑✟☛✱✒❢❨✦✔❆✦▲✲❈❁✁❃❍✶✵❣✮❫❁✘☞✠✡✓✞✑✟✠✡✎➳➸❺❑❁✁❃✳❃✣✤❃❉❁✔✲✴✐❤❝✱▲❥ |
|
||||||||||||||
|
✷❇✱❂❦✤❆✠✷✻ ➶✔❄✸❅✄❆✖✷❂✱✳✲▼✴✞❒✌✙✘☞☛✞✫❉✠✙✄✦➮ |
|
|
|
|
|
|
|
||||||||
|
✎✏☛✞✄➨✲✭✄☞☛✞✓✞✄✆✁✵✰✳✎➣✃➣✑❢✘☞✬❚✰❛✘☞☛✍☛✱✝➆➮✪➱✈✒✯✮✗✰✞✕✗✍✔❧✁❧ ✶✵➽❆◆❅❑✰✔❄❉❅✄❆✖✷❂✱✳✲✖✴✯✽▼✻❏✕✗✍✔✍✠✕✞➮ |
|
|
|
||||||||||||
✦➮✏ ☛☞☛✞✝➐✢▲✫❉✠✙✄☞✓✐✁✵✒✴✢✹✠✫❍✠✙✒✔ ✠✙✎✏✎❃✶✍➮✪ |
|
|
|
|
|
|
|
|||||||||
✙❝✦❛➮❲☞❍✩✔✲▲✘✆✁▲❒❭❍➭➮❵❍❚✁✵✄✏✘☞☛✞✒✔☛✱✎✏✑➆❒✿✁✞✒✔✢➲➹❍➮❲✦❮✒✴✢▲✠✙✄☞✎✏☛✱✒✗➮✌❍❰✠✙✰✳✎✏✲➨✒✴✁✞✝❢✶▲➴✡✑✟✒✔✓❰✰✳✠✡✰✳☛✞✄✏✶♠☎✼✦ |
|
|||||||||||||||
✦✓ |
✛✫❉☛✞✘✏✘☞✝✟✠✡✒✭✌♣➬✦✎❧✑✟✒❚✲✭✄☞☛✞✓✞✄✆✁✵✰✳✎✡➮✠✩✐➮●✩③✁✵✲✔✝✟☛✉✃❮➮☛✩✐➮✴✤✦➴✙✶▲✰✹✁✞✒✭✎✏➬▲✑➆➮✪ |
|
|
|
|
|
|
|||||||||
|
|
|
|
|
|
♥✮➨✺✵▲❄❙✔✲✄✰➻✲❈❁✁❃➪❇♦✗✱✼ ♠✒✢✛✭➫✶✕☎➯♣✕✗✍✔✍✑✒☎❒✦➮ |
|
|
|
|
||||||
|
✌✉✁✞✌✆✬✔✠★✲❉✠♣✄✏➸❺☛✞✄☞✰✹✁✵✒✔✌✡✠★✲▲✄☞✧✮❑❁✁❃✺❃✤❃❈✮✗✰▲✱✺✲✖✴❚❘❇✷❂✷✉✢✭✑✟✌✙✘☞✑✟☛✞✒◆➮✻❖✙✭➫✶✕✉➯❇☎ ✭❒❵✕✚✍✁✍✁✙✦➮ |
|
||||||||||||||
▲➮❧✞❍➭➮✗✤❊➮❉✕➚✁✞✰❚❒❉➺➣➮✗➺❧➮❉➧➨☛✞✘☞✬✦✫❉✙✄☞✓✭❒✗✁✵✒✴✢✛☛✱✝❢➸✈➮❿➹➘✬✔✠➳✮➘✁✞✌✆✬✔✠❈☛✱✲▲✘☞✑✟✰✳✑✣➴✡✁✵✘☞✑✟☛✱✒❚✫✔✁✞✎✏✠✉✢✖☛✱✒❪✌✡☛✱✰✳✲✭✑✣✝✟✠✙➷❼✘☞✑✟✰✳✲✭✘☞✑✟✰✳✑✟➴✉✁✵➷ |
||||||||||||||||
|
✘☞✑✟☛✱✒✔✎✹☛✵➸❬✟✯✝✟☛✍✌♣➬✱✠✉✢❡✦➨✝✣✓✞☛✞✄☞✑❢✘☞✬✔✰✳✎✡➮➱✈✒✉✮✗✰◆✈➻❛➶❏■➻❍➽✮✬❘❳➽➽❁✔✲☛✷❜❁❃✇❁❑❁✔❳➶ ➶➚➻❒➓✲✔✁✞✓✱✠✙✎ |
|
||||||||||||||
|
✃➣✑❢✘☞✬❁✝✟☛✍☛✱✲❁✘✏✄✆✁✵✒✔✎❃➸↔☛✞✄☞✰✹✁✵✘☞✑✟☛✞✔✎✡➮★✑✣✒✭✝✟✠✙✶✍❒➚✤✦✘☞✠✡➩❴✠✐✮➘✁✵✄✏✄✉❒➻❛➶❏■➾❑❁✔✲✤❁✁✰✤✷❂✱❼✔✲✔✲✪✮✠✙✒➭➹➘✎✏✠✙✒✔✓✭➮➳➱✈✰✳✲▲✄☞☛✵➩✦✑✟✒✔✓✖✢✭✁✵✘✆✁❪✝✟☛✍✌✉✁✵✝✟✑✟✘✻✶❧✞❑❁✔❄❀❄◗✱✳✲▼✴❱❘♣❁✔✲❈❁✗✴✔❆☛❁✗✴✌❁✔✲❉ |
|||||||||||||||
|
✙✁✜✗❊✠✓✢❋▲➮♥✦➨✮✝❍➭❒✔➵❉①➳❒☛✦➨✲✭✄☞✑✟✝❵✕✗✍✁✍✖✕✱➮ |
|
✛ |
|
|
|
|
|
|
|||||||
▲➮✍✸✩③✁✵✘☞✬▲✄✏✶✭✒➭✤❉➮❲❍❰✌✤✩ |
|
|
|
|
|
|
|
|
|
|
||||||
✦➮✗✛✞✩③✵✘☞✬▲✄✏✶✭✒♥❍❰✌✚✩★✑✟✒✔✝✟✠✙✶✝✟✑✟➩❴✠✙✄❰➹➚✠✡✰✹✁✵✰❚➮②✦❐✍✩✴✁✵✒✍✘✆✁✵✘✆✁✵✘☞✑✟➩❴✠ |
|
|
||||||||||||||
|
➽✻❇❄➳❏✕✚❧✭➫❂❋✍➯❇☎❋✑✒✢❋✢❊✑❋▼✏✢✜▲❒◆❩✕✗✍✔✍✁✙▲➮ |
|
|
|
|
|
|
|
||||||||
|
✝✟☛✍✌✉✁✞✝✟✑❢✈✶✍➮➓➱✈✒➻❿➽✮✧❘✝❯❥◆③✄✦➽ ❪❑❪➨✮✝❍➭❒❖✕✗✍✁✍✔✙▲➮ |
|
|
|
|
|
|
|
||||||||
|
✌✡☛✱✰✳✲✭✩✭✘✆✁☎✘☞✑✣✦✔❆✦▲✲❈❁✁❃➚✶✵❫✮❫❁❑❁✁❃✳❃✤❃☛✞✝✣✠✙✎✏✒◆➮ ➻ |
❴▲✱✳✷✺✹✖❄➳✞❁✔✲❉➻❅✁❅❈❃④✰❼✔✲✭♠✒▲➫✶✕✉➯❇☎✏✗❊✠✒✢✙▲❒❲✕✚✍✁✍✢❋✭➮ |
|
|||||||||||||
✞✁✕❬✟➘✁✞✝❢✄✆✁✵✰✤▲✑✟✒✭✬✴✁✵✄☞✉✶✯ |
✤▲➴♣✶✭✰✹✁✵✒✔✎✏➬✦✑➆➮➐✁✡✃ ❉✡ |
✃➘✁✉➩❴✠✙➸↔✄☞☛✞✒✍✘★☛✞➸✿✲✴✁☎✄✆✁✞✝✟✝✟✠✡✝ |
||||||||||||||
|
✰✹✁✵ |
|
|
|
|
|
❪✶⑤✬⑤✧⑤➾❑❁✔✲✚✈❱✮❫❁❑❁✁❃✳❃✝✟☛✍☛✞✲❵✘✏✄✆✁✞✒✭✎❃➸❺☛✵✄☞✰✹✁✵✘☞✑✟☛✱✒❨✘☞✬✔✠✡☛✵✄✏✶❨✁✞✒✴✢❵✁✞✒❵✁✞✝✟✓✱☛✵✄☞✣✤❃♥❁✔✲❉✯❤✞✱❺➆▲✱④❦✤❆✖✷✻ ➽▲✷✻❇❄❈⑥✜▲➫✶✕✗✛✱➯❇☎❋✑✏✁✒✚❊ |
|||||||||
✕✍◆✒✼❍➮➭➮✿➺➣➮☛✞✝❢➸★✁✞✒✔✢✛ ❡❍➭➮➘✤❊➮➓✕◆✁✞✰❚➮❡✦ |
|
|
|
|
|
✘☞☛ |
|
|||||||||
|
❋▼✓▼✕✱❒❏✕✚✍✁✍✖✕✱➮ |
|
|
|
|
✿✁ |
|
|
|
|
|
|
|
|||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|