Delving into the realm of trigonometry, the Three Angle Measure Introduction form unfolds as an essential building block for understanding complex mathematical concepts. This form is a pivotal part of the curriculum, designed to equip students with the foundational skills necessary for mastering trigonometry. It serves to connect theoretical knowledge with practical application through a series of carefully curated problems and exercises detailed in Lesson 8.1 Skills Practice. The form is structured to enhance the learner's proficiency in identifying relationships between angles and sides within triangles, thereby laying a solid groundwork for trigonometric ratios. Through tasks that involve determining ratios like opposite to hypotenuse and adjacent to hypotenuse, using given reference angles in diverse triangles, students are guided towards crafting their answers in the simplest fractional form. This meticulous approach not only aids in honing their problem-solving techniques but also in appreciating the elegance and utility of trigonometry in mathematical discourse and its applications beyond the classroom.
| Question | Answer |
|---|---|
| Form Name | Three Angle Measure Introduction Form |
| Form Length | 42 pages |
| Fillable? | No |
| Fillable fields | 0 |
| Avg. time to fill out | 10 min 30 sec |
| Other names | Secant, Cotangent, three angle measure introduction to trigonometry 9 1 answers, Cosines |
LESSON 8.1 |
Skills Practice |
8 |
|
|
|
Name |
|
Date |
Three Angle Measure
Introduction to Trigonometry
Vocabulary
Use the diagram to complete each sentence.
1. |
If b is the opposite side, then x is the |
|
. |
|
|
|
|
|
|
|
|
2. |
If y is the reference angle, then b is the |
|
. |
||
3. |
If x is the reference angle, then b is the |
|
. |
||
Y
C
b
X
A
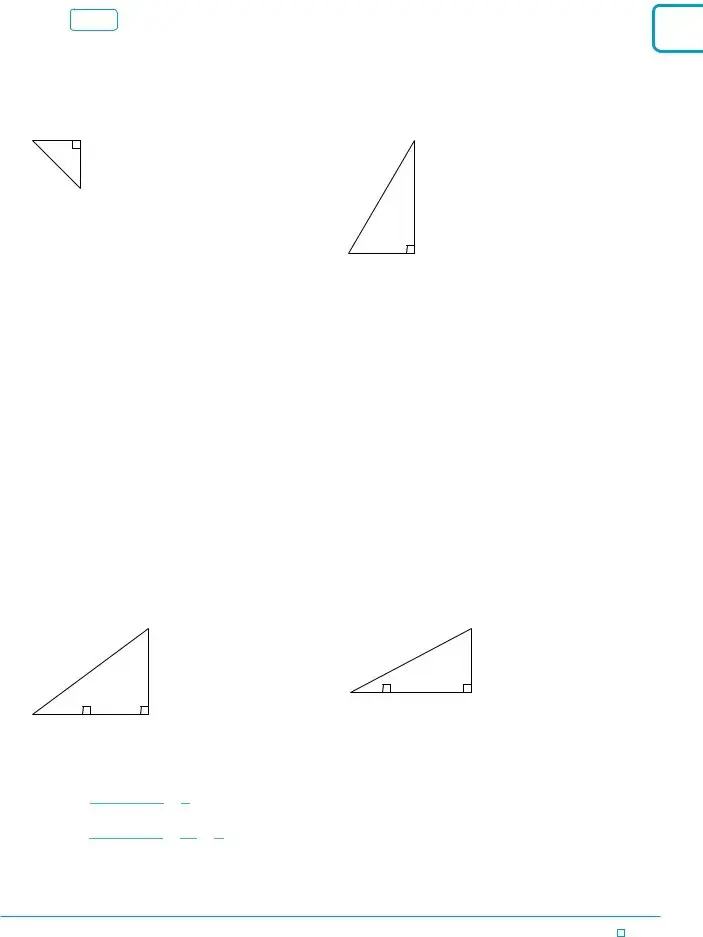
Problem Set
Determine the ratio |
opposite |
|
||||
___________ using /A as the reference angle in each triangle. Write your answers as |
||||||
|
|
|
|
hypotenuse |
|
|
fractions in simplest form. |
|
|
||||
1. |
|
B |
|
|
2. |
B |
|
10 |
|
6 |
|
|
|
|
|
|
|
|
|
|
A |
8 |
C |
|
|
|
|
|
|
|
|
|
26 |
24 |
|
|
|
|
|
A 10 |
C |
|
opposite |
|
5 |
6 5 |
3 |
|
hypotenuse |
|
10 |
5 |
|
||
© Carnegie Learning
Chapter 8 SKILLS PRACTICE |
685 |
8 |
LESSON 8.1 Skills Practice |
|
PAGE 2
3. |
B |
4. |
B |
7
A 24C
15
A 8 C
5. B |
6. B |
12
√3
C 9 A
C 1 A
|
|
ad |
|
|
|
Determine the ratio ___________ using /A as the reference angle in each triangle. Write your answers as |
|||||
|
|
hypotenuse |
|
|
|
fractions in simplest form. |
|
|
|
||
7. |
|
B |
8. |
|
B |
|
25 |
15 |
|
|
|
|
|
|
|
|
|
|
|
|
|
34 |
30 |
|
|
|
|
|
|
A |
20 |
C |
|
|
|
A 20 C
adjacent 20 4 hypotenuse 5 25 5 5
© Carnegie Learning
686 
Chapter 8 SKILLS PRACTICE
LESSON |
8.1 |
Skills Practice |
|
|
PAGE 3 |
8 |
||
|
|
|
|
|
|
|
|
|
Name |
|
|
|
|
Date |
|
|
|
9. |
|
|
B |
10. A |
|
|||
|
|
|
1.4 |
|
|
|
|
|
A |
4.8 |
C |
|
|
|
|
|
|
|
|
|
|
4 |
|
|
|
|
C 4B
© Carnegie Learning
11. |
B |
12. A |
2√ |
3 |
C |
2
B
2.4
C 1.0 A
Chapter 8 SKILLS PRACTICE |
687 |
8 |
LESSON 8.1 Skills Practice |
|
PAGE 4
opposite |
ad |
opposite |
Determine the ratios ___________, ___________, and _________ using /A as the reference angle in each |
||
hypotenuse |
hypotenuse |
ad |
triangle. Write your answers as fractions in simplest form.
13. |
|
|
|
B |
|
|
30 |
|
|
|
|
|
|
|
|
18 |
|
A |
24 |
|
|
C |
|
|
opposite |
5 |
18 |
5 |
3 |
hypotenuse |
|
30 |
|
5 |
|
|
adjacent |
5 |
24 |
5 |
4 |
hypotenuse |
|
30 |
|
5 |
|
|
opposite |
5 |
18 |
5 |
3 |
|
adjacent |
|
24 |
|
4 |
15.A
51
C 24 B
14.B
1.3
0.5
C 1.2 A
16. A |
20 |
C |
29
B
© Carnegie Learning
688 
Chapter 8 SKILLS PRACTICE
LESSON |
8.1 Skills Practice |
|
|
|
PAGE 5 |
8 |
||||
|
|
|
|
|
|
|
|
|
|
|
Name |
|
|
|
|
|
|
Date |
|
|
|
17. A |
C |
18. |
B |
|
||||||
|
|
|
|
5 |
|
|
|
|
|
|
5√2 |
|
|
|
|
|
|
||||
|
|
|
|
|
|
|
||||
B |
12 |
A 6 C
© Carnegie Learning
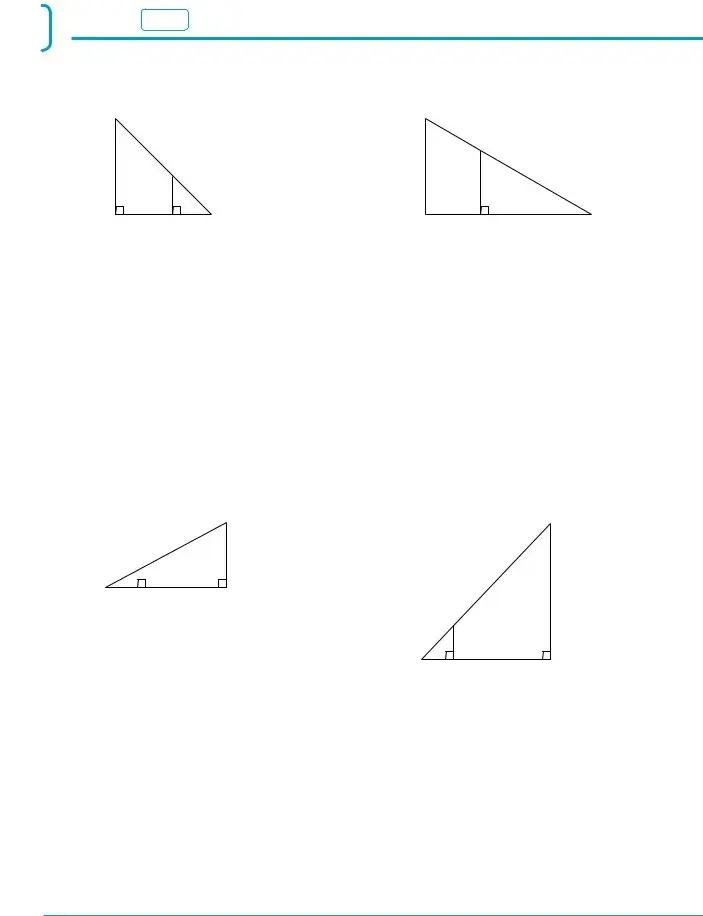
In each igure, triangles ABC and DEF are similar |
AA em. Calculate the indicated |
|||||||||||
ratio twice, irst using NABC and then using NADE. |
|
|
|
|
|
|
||||||
|
opposite |
|
|
|
|
ad |
|
|
|
|
||
19. |
___________ for reference angle A |
20. |
___________ for reference angle A |
|||||||||
|
hypotenuse |
|
|
|
|
hypotenuse |
|
|
||||
|
|
|
D |
|
|
|
|
|
|
|
|
D |
|
|
5 |
|
|
|
|
|
|
|
34 |
|
|
|
|
B |
|
|
|
|
|
B |
|
24 |
||
|
|
|
6 |
|
|
|
|
17 |
|
8 |
|
|
|
|
|
|
|
|
|
|
|
|
|
||
|
5 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
3 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
A 15 C |
30 |
E |
|||
|
|
|
|
|
|
|
|
|||||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
A 4 |
C 4 E |
|
|
|
|
|
|
|
|
|
|
|
AE 5 4 1 4 5 8 |
|
|
|
|
|
|
|
|
|
||
|
AD 5 5 1 5 5 10 |
|
|
|
|
|
|
|
|
|
||
|
In NABC, |
|
opposite |
5 |
3. |
|
|
|
|
|
|
|
|
|
|
hypotenuse |
|
5 |
|
|
|
|
|
|
|
|
In NADE, |
|
opposite |
5 |
6 |
5 3. |
|
|
|
|
|
|
|
|
hypotenuse |
|
10 |
5 |
|
|
|
|
|
|
|
Chapter 8 SKILLS PRACTICE |
689 |
8 |
LESSON 8.1 Skills Practice |
|
PAGE 6
|
opposite |
|
|
|
|
ad |
|
|
|
||||
21. |
___________ for reference angle A |
22. |
___________ for reference angle A |
||||||||||
|
hypotenuse |
|
hypotenuse |
|
|
|
|||||||
|
D |
|
|
|
|
D |
|
|
|
||||
|
|
|
|
|
|
|
|
2 |
|
|
|
||
|
15√2 |
|
|
|
|
|
|
B |
|
|
|
||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
25 |
|
|
B |
|
3 |
|
|
4 |
|
|||
|
|
|
|
|
|
|
|
|
|
|
|
||
|
|
|
|
|
|
|
|
2 |
|
|
|
||
|
10 |
10√2 |
|
|
|
|
|
|
|
||||
|
|
|
|
|
|
|
|
|
|
|
|||
|
|
|
|
E √ |
|
C |
2√ |
|
|
||||
|
E 15 |
C 10 A |
|
3 |
3 |
A |
|||||||
opposite
23. ________ad for reference angle A
D
34
opposite
24. ________ad for reference angle A
D
B24
17 |
|
8 |
|
8.7 |
|
|
|
|
|
||
|
|
|
|
8.4 |
|
A 15 C |
30 |
E |
|||
|
|||||
B
2.92.1
A 2.0 C |
6.0 |
E |
© Carnegie Learning
690 
Chapter 8 SKILLS PRACTICE
LESSON 8.2 |
Skills Practice |
8 |
|
|
|
Name |
|
Date |
The Tangent Ratio
Tangent Ratio, Cotangent Ratio, and Inverse Tangent
Vocabulary
esponding term for triangle |
EFG. |
F
|
E |
G |
|
1. |
EG in relation to /G |
A. tangent |
|
|
EF |
|
|
2. |
EF in relation to /G |
B. cotangent |
|
EG |
|
3. tan2 |
EGEF ) in relation to /G |
C. in |
© Carnegie Learning
Chapter 8 SKILLS PRACTICE |
691 |
8 |
LESSON 8.2 Skills Practice |
PAGE 2 |
|
|
Problem Set
Calculate the tangent of the indicated angle in each triangle. Write your answers in simplest form.
1. |
2 FT |
B |
2. 3 2 FT |
|
|
|
|
|
|
|
2 FT |
|
|
3 2 FT |
|
|
|
|
B |
|
tan B 5 |
2 |
5 1 |
tan B 5 |
|
|
2 |
|
|
3. |
4. |
C |
|
25 M |
|
|
|
40 M |
C |
|
|
20 M |
|
32 M |
|
|
|
tan C 5 |
|
tan C 5 |
5. |
15 M |
6. |
3 FT |
D
2 2 M
5 5 FT
D
tan D 5 |
tan D 5 |
© Carnegie Learning
692 
Chapter 8 SKILLS PRACTICE
© Carnegie Learning
LESSON 8.2 Skills Practice |
|
PAGE 3 |
8 |
||
|
|
|
|
|
|
Name |
|
Date |
|
|
|
Calculate the cotangent of the indicated angle in each triangle. Write your answers in simplest form.
7. |
|
|
|
|
|
|
8. |
A |
|
|
|
|
|
|
3 ft |
|
|
|
|
|
|
|
|
|
|
|
|
6 FT |
|
|
A |
|
4 ft |
|
|
|
|||
|
|
|
|
|
|
||||
|
|
|
|
|
|
|
|
8 FT |
|
|
|
4 |
|
|
|
|
|
||
|
cot A 5 3 |
|
cot A 5 |
|
|||||
9. |
|
|
|
|
|
F |
10. |
|
|
|
|
|
|
|
|
7 yd |
|
6 yd |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
15 yd |
|
|
|
|||
|
|
|
|
|
|
|
|
2 6 yd |
F |
|
|
|
|
|
|
|
|
|
|
|
cot F 5 |
|
cot F 5 |
|
|||||
11. |
|
4√ |
|
FT |
12. |
32 M |
|
||
|
2 |
A |
|||||||
|
|
|
|
|
|
|
|
|
|
|
|
|
|
4√ |
|
FT |
|
|
|
|
|
|
|
2 |
|
|
|
||
|
|
|
|
|
|
|
|
40 M |
|
|
|
|
|
A |
|
|
|
||
|
cot A 5 |
|
cot A 5 |
|
|||||
Use a calculator to approest hundredth. |
|
|
|
||||||
13. |
tan |
|
|
|
|
|
14. |
tan |
|
|
0.58 |
|
|
|
|
|
|
|
|
15. |
tan |
|
|
|
|
|
16. |
tan |
|
17. |
tan |
|
|
|
|
|
18. |
tan |
|
Chapter 8 SKILLS PRACTICE |
693 |
8 |
LESSON 8.2 |
Skills Practice |
|
PAGE 4 |
|
|
|
|
|
|
|
|
Use a calculator to approest hundredth. |
|
|
||
|
19. |
cot |
|
20. |
cot |
|
|
0.58 |
|
|
|
|
21. |
cot |
|
22. |
cot |
23. cot |
24. cot |
Use a tangent ratio or a cotangent ratio to calculate the missing length of each triangle. answers to the nearest hundredth.
25. |
2 ft |
26. |
X |
|
40° 
X |
6 ft |
|
|
|
60° |
tan 40° 5 2X
2 tan 40° 5 X
X < 1.68 ft
27.28.
15 m5°
20° |
|
X |
|
|
|
||
X |
|||
|
|||
2 M
© Carnegie Learning
694 
Chapter 8 SKILLS PRACTICE